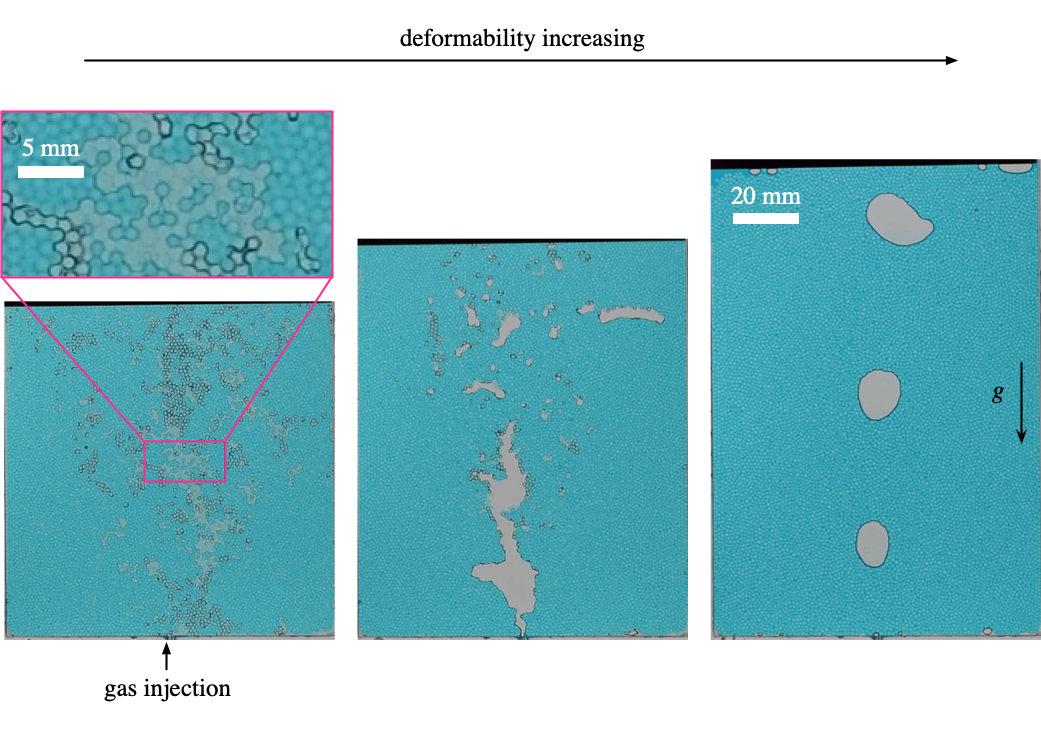
Figure 1. Upward injection of non-wetting gas (light color) into a vertical, quasi-2D porous medium saturated with liquid (blue). Under a large confining stress (left), the medium is weakly deformable and the gas migrates through the pore space without changing the structure of the medium. As the confining stress decreases (middle and right), the medium is increasingly deformable and the pore structure exhibits increasingly dramatic changes in response to the motion of the gas. Adapted from [1].

The scenario described above is one where deformation is driven by the viscous pressure gradient and resisted by elasticity. In other scenarios, different definitions of deformability may be more appropriate. In the presence of two fluid phases, for example, the capillary pressure might better capture the typical size of pressure variations; the relevant pressure scale would then be 𝛿p ~ 𝛾/d for interfacial tension 𝛾 and pore size d. In a ductile medium, the shear strength and/or the confining stress might be a more relevant measure of the resistance to deformation. In general, the importance of deformation should be assessed by comparing the strongest relevant driving force with the weakest relevant resistance.

No material is perfectly rigid, but deformation can usually be neglected in problems where the deformability is very small. Although many challenges and open questions remain, our basic physical understanding and theoretical descriptions of flows through rigid porous media are well established and widely used. Flow and transport through rigid porous media are typically described by conservation of mass, Darcy’s law, and macroscopic flow properties such as permeability, relative permeability, capillary pressure, and hydrodynamic dispersivity. It is widely agreed that these flow properties are characteristic of the particular pore structure of the medium.

In a “stiff” porous medium, the deformability is small but not negligible. In these weakly deformable systems, the main impact of deformation is through the coupling of pressure with stress, which leads to small changes in pore volume. The well-known physical manifestation of these changes is the development of diffusive pressure transients, as described by the classical theory of linear poroelasticity. Linear poroelasticity has been widely and successfully used in geomechanics and hydrology as a tool for understanding pressure diffusion in the subsurface due to injection, pumping, and surface loading [e.g., 2-4].

In stiff porous media, deformations are assumed to have a negligible impact on pore structure. Because the pore structure is preserved, the macroscopic flow properties of the medium are assumed to remain unchanged. Note, however, that changes in pore structure can result from physical mechanisms other than fluid pressure. For example, sufficiently large changes in external confining stress can lead to moderate deformations even when the flow itself cannot, and these deformations can have a strong impact on pore structure and therefore also on flow properties [e.g., 5-6]. This situation involves a one-way mechanical coupling between deformation and flow, in the sense that the deformation changes the flow but not vice versa. Other processes, such as chemical reaction and phase change, can lead to moderate or even large changes in pore structure that exhibit a two-way coupling with flow via the additional role of solute or heat transport; however, these processes do not involve mechanical deformation in the sense that we mean it here.

As defined above, the deformability is a measure of the strain experienced by the solid in response to interactions with the fluid — that is, D measures the deformability of the skeleton. The deformability of the pore space is better measured by the associated fractional change in pore volume, which can be estimated as ((1-ϕ0)/ϕ0)D, where ϕ0 is the undeformed porosity. As a result, the above reasoning about stiff porous media is incorrect in situations where ϕ0 is very small, as in fractured rocks such as granite, chalk, or coal. In those cases, small strains in the solid (i.e., a weakly deformable skeleton) can correspond to large changes in the pore structure (i.e., a highly deformable pore space). An appropriate theory for flow through deformable, low-porosity materials should account for deformation-dependent flow properties, even when otherwise linearised in the strain.

In a “soft” porous medium, the deformability is moderate or even large. The defining feature of these highly deformable systems is the strong coupling between the fluid flow and the structure of the pore space. For example, the pores may become isotropically smaller, as when squeezing a sponge, or they may become elongated along one axis and constricted along another, as when stretching a tissue or fabric, or they may be destroyed and reformed as the grains flow past and around one-another, as when shearing a sediment. “Softness” may arise due to a very low elastic stiffness, as in biological tissues, biofilms, gels, and textiles, or due to brittleness and/or ductility, as in soils and sediments, glaciers and ice sheets, and partially molten metals and rocks.

One clear challenge of flows through soft porous media is in describing the kinematics and rheology of the solid skeleton during large deformations. For example, highly elastic materials such as gels and biological tissues are typically described by nonlinear elasticity, whereas highly ductile materials such as soils and sediments usually require nonlinear elasto-plasticity. Substantial bodies of literature in biomechanics and geomechanics are dedicated to capturing these complexities and, to a lesser extent, their interaction with the redistribution of pore fluid.

Softness also has profound implications for the transport and mixing of solutes, and for the interaction of multiple fluid phases. These phenomena have attracted some attention in the context of fluid-driven deformation patterns and fracturing [e.g., 1,7,8]. In general, however, the strong interaction between deformation and flow properties that occur in soft porous media remain at the frontier of our ability to measure and model.

This work was supported by the European Research Council (ERC) under the European Union's Horizon 2020 Programme (Grant No. 805469).