PORE 1-2: Introduction to Single Phase Flow and Transport in Porous Media
This course aims to provide a foundational understanding of flow and transport in porous media, emphasizing its broad applications across various fields, including energy, hydrology, geothermal systems, and the emerging areas of carbon sequestration and hydrogen storage.
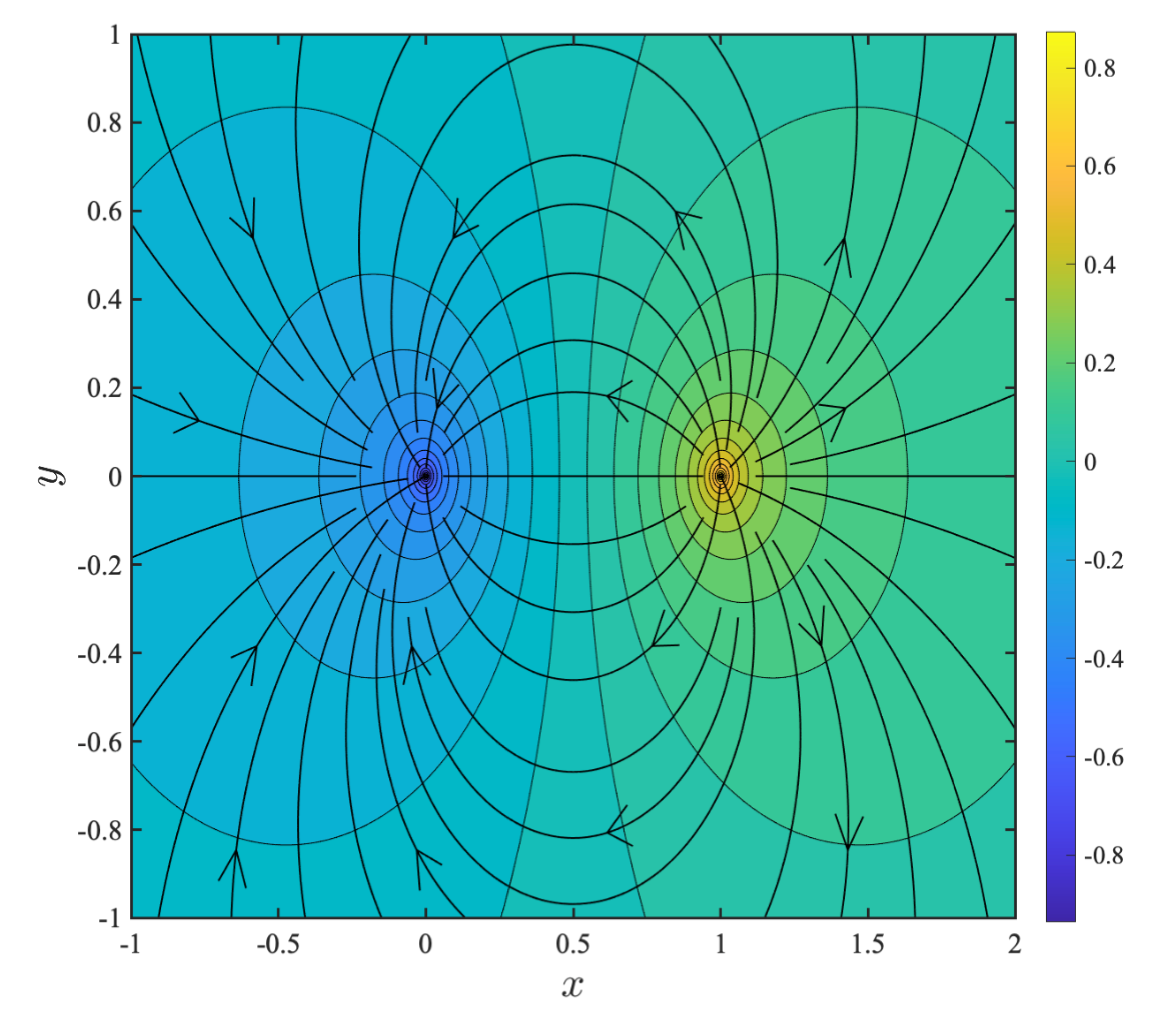
The course is an attempt to present the fundamentals of flow and transport in porous media, motivated by its applications in many fields, including energy, hydrology, geothermal, and, more recently, carbon sequestration and hydrogen storage. The primary goal is to provide tools to understand what’s going on “behind the scenes” of the continuum description of flow in porous media. Starting from first principles, we will go through the microscopic and macroscopic levels of description, introducing the concept of representative elementary volume. Then, we will present the equation of motion, starting from the classical Darcy’s experiment and reviewing its generalization to three-dimensional flow. Analytical solutions to the equation of motion will be used to get familiar with the estimation of fluxes in homogenous materials. Finally, we will describe the fundamentals of hydrodynamic dispersion (also known as Aris-Taylor dispersion), namely the spreading of a scalar in a porous medium. First, we recall that a scalar can be transported through two fundamentally different mechanisms (advection and diffusion), discussing the key features of the one-dimensional advection diffusion equations. Then we will look into the classical theory of Aris-Taylor and discuss its ramifications for practical applications.